Cho hàm số f(x)=ax4+bx3+cx2+dx+e,(a≠0)f(x)=ax4+bx3+cx2+dx+e,(a≠0). Hàm số f′(1−x) có đồ thị
Cho hàm số f(x)=ax4+bx3+cx2+dx+e,(a≠0). Hàm số f′(1−x) có đồ thị như hình vẽ bên dưới.
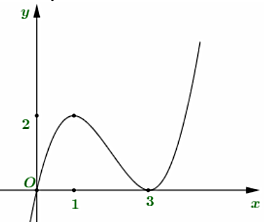
Tìm số điểm cực trị của hàm số g(x)=f(x2−1x2)−x2
Quảng cáo
Ta có: g(x)=f(x2−1x2)−x2=f(1−1x2)−x2.
g′(x)=(1−1x2)′f′(1−1x2)−2x=2x3f′(1−1x2)−2x.
g′(x)=0⇔2x3f′(1−1x2)=2x⇔f′(1−1x2)=1(1x2)2(∗).
Nhận xét: Số cực trị của hàm số g(x)=f(x2−1x2)−x2 là số giao điểm (không tính điểm tiếp xúc) của đồ thị hàm số y=f′(1−1x2) và đồ thị hàm số y=1(1x2)2. Vẽ đồ thị hàm số h(x)=1x2 trên cùng một hệ trục với đồ thị hàm số y=f′(1−x), ta được:
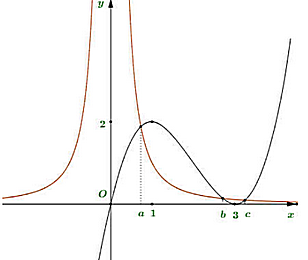
(∗)⇔[1x2=a∈(0;1)1x2=b∈(1;3)1x2=c∈(3;+∞)⇔[x2=1ax2=1bx2=1c⇔[x=±√1ax=±√1bx=±√1c
Vậy hàm số có 6 cực trị
>> Lộ Trình Sun 2025 - 3IN1 - 1 lộ trình ôn 3 kì thi (Luyện thi TN THPT & ĐGNL; ĐGTD - Click xem ngay) tại Tuyensinh247.com. Đầy đủ theo 3 đầu sách, Thầy Cô giáo giỏi, 3 bước chi tiết: Nền tảng lớp 12; Luyện thi chuyên sâu; Luyện đề đủ dạng đáp ứng mọi kì thi.
 |
 |
 |
 |
 |
 |
 |
 |
Hỗ trợ - Hướng dẫn

-
024.7300.7989
-
1800.6947

(Thời gian hỗ trợ từ 7h đến 22h)
Email: lienhe@tuyensinh247.com












