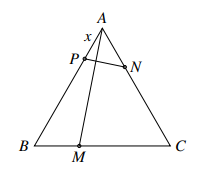
Cho tam giác ABC đều cạnh 3a. Lấy M, N, P lần lượt nằm trên ba cạnh BC, CA, AB sao cho

Câu hỏi số 725968:

Vận dụng

\frac{1}{3}

$\dfrac{1}{3}$

\frac{4}{5}

$\dfrac{4}{5}$

\frac{2}{3}

$\dfrac{2}{3}$

\frac{x}{3 a}

$\dfrac{x}{3a}$

\frac{4}{3}

$\dfrac{4}{3}$

Cho tam giác ABC đều cạnh 3a. Lấy M, N, P lần lượt nằm trên ba cạnh BC, CA, AB sao cho $BM = a,CN = 2a,AP = x(x > 0)$ .

a) $\overrightarrow {AM} =$ $\overrightarrow {AB} +$ $\overrightarrow {AC}$ và $\overrightarrow {NP} =$ $\overrightarrow {AB} - \dfrac{1}{3}\overrightarrow {AC}$

b) $AM \bot NP \Leftrightarrow x =$ $a$

Đáp án đúng là: $\dfrac{2}{3}$ , $\dfrac{1}{3}$ , $\dfrac{x}{3a}$ , $\dfrac{4}{5}$

Quảng cáo

Xem lời giải

Câu hỏi:725968

Giải chi tiết

a) Ta có: $\overrightarrow {BC} = 3\overrightarrow {BM} \Rightarrow \overrightarrow {AC} - \overrightarrow {AB} = 3(\overrightarrow {AM} - \overrightarrow {AB} )$

$\Rightarrow \overrightarrow {AM} = \dfrac{2}{3}\overrightarrow {AB} + \dfrac{1}{3}\overrightarrow {AC}$

Mặt khác: $\overrightarrow {NP} = \overrightarrow {AP} - \overrightarrow {AN} = \dfrac{x}{{3a}}\overrightarrow {AB} - \dfrac{1}{3}\overrightarrow {AC}$ .

b) Ta có: $\overrightarrow {AB} \cdot \overrightarrow {AC} = \dfrac{{9{a^2}}}{2}$ .

$\begin{array}{l}AM \bot NP \Rightarrow {\rm{ }}\overrightarrow {AM} .\overrightarrow {NP} = 0\\ \Leftrightarrow \left( {\dfrac{2}{3}\overrightarrow {AB} + \dfrac{1}{3}\overrightarrow {AC} } \right)\left( {\dfrac{x}{{3a}}\overrightarrow {AB} - \dfrac{1}{3}\overrightarrow {AC} } \right) = 0\\ \Leftrightarrow \dfrac{{2x}}{{9a}}A{B^2} - \dfrac{2}{9}\overrightarrow {AB} .\overrightarrow {AC} + \dfrac{x}{{9a}}\overrightarrow {AC} .\overrightarrow {AB} - \dfrac{1}{9}A{C^2} = 0\\ \Leftrightarrow \dfrac{{2x}}{{9a}} \cdot {(3a)^2} - \dfrac{2}{9} \cdot \dfrac{{9{a^2}}}{2} + \dfrac{x}{{9a}} \cdot \dfrac{{9{a^2}}}{2} - \dfrac{1}{9} \cdot {(3a)^2} = 0\\ \Leftrightarrow x = \dfrac{{4a}}{5}\end{array}$

Group 2K8 ôn Thi ĐGNL & ĐGTD Miễn Phí

Câu hỏi trước Câu tiếp theo

>> Lộ Trình Sun 2025 - 3IN1 - 1 lộ trình ôn 3 kì thi (Luyện thi TN THPT & ĐGNL; ĐGTD - Click xem ngay) tại Tuyensinh247.com. Đầy đủ theo 3 đầu sách, Thầy Cô giáo giỏi, 3 bước chi tiết: Nền tảng lớp 12; Luyện thi chuyên sâu; Luyện đề đủ dạng đáp ứng mọi kì thi.