Trong mặt phẳng với hệ trục OxyOxy, cho bốn điểm A(1;3)A(1;3), \(B\left( {2; - 1}
Trong mặt phẳng với hệ trục OxyOxy, cho bốn điểm A(1;3)A(1;3), B(2;−1)B(2;−1), C(−3;−2)C(−3;−2), M(3;4)M(3;4) và điểm PP thay đổi thỏa mãn →PA.→PB+→PB.→PC+→PC.→PA+2=0−−→PA.−−→PB+−−→PB.−−→PC+−−→PC.−−→PA+2=0. Tìm giá trị nhỏ nhất của MPMP.
Đáp án đúng là: B
Quảng cáo
- Gọi P(x;y)P(x;y). Tính →PA.→PB+→PB.→PC+→PC.→PA−−→PA.−−→PB+−−→PB.−−→PC+−−→PC.−−→PA.
- Tìm tập hợp các điểm PP, từ đó tìm GTNN của MPMP.
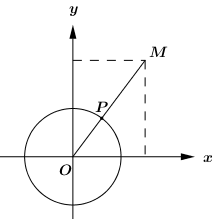
Gọi P(x;y)P(x;y).
Ta có →PA=(1−x;3−y)−−→PA=(1−x;3−y); →PB=(2−x;−1−y)−−→PB=(2−x;−1−y); →PC=(−3−x;−2−y)−−→PC=(−3−x;−2−y).
⇒→PA.→PB=(1−x)(2−x)+(3−y)(−1−y)=x2+y2−3x−2y−1→PB.→PC=(2−x)(−3−x)+(−1−y)(−2−y)=x2+y2+x+3y−4→PC.→PA=(−3−x)(1−x)+(−2−y)(3−y)=x2+y2+2x−y−9⇒→PA.→PB+→PB.→PC+→PC.→PA+2=0⇔3x2+3y2−12=0⇔x2+y2=4
⇒ Tập hợp các điểm P là đường tròn tâm O(0;0) bán kính R=2.
Vậy MPmin=OM−R=√32+42−2=3.
Chọn B.
>> Lộ Trình Sun 2025 - 3IN1 - 1 lộ trình ôn 3 kì thi (Luyện thi TN THPT & ĐGNL; ĐGTD - Click xem ngay) tại Tuyensinh247.com. Đầy đủ theo 3 đầu sách, Thầy Cô giáo giỏi, 3 bước chi tiết: Nền tảng lớp 12; Luyện thi chuyên sâu; Luyện đề đủ dạng đáp ứng mọi kì thi.
 |
 |
 |
 |
 |
 |
 |
 |
Hỗ trợ - Hướng dẫn

-
024.7300.7989
-
1800.6947

(Thời gian hỗ trợ từ 7h đến 22h)
Email: lienhe@tuyensinh247.com












