Đánh giá năng lực Hà Nội
Đánh giá năng lực Hà Nội
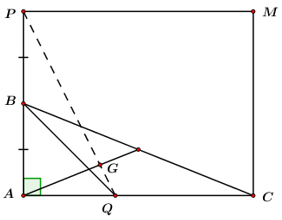
Cho ΔABCΔABC vuông tại AA có độ dài các cạnh AB=2cm,AC=5cm.AB=2cm,AC=5cm. Gọi PP là điểm
Cho ΔABCΔABC vuông tại AA có độ dài các cạnh AB=2cm,AC=5cm.AB=2cm,AC=5cm. Gọi PP là điểm đối xứng với AA qua B;B; điểm QQ trên cạnh ACAC sao cho AQ=25AC.AQ=25AC.
a) Chứng minh rằng 5→PQ+10→AB−2→AC=→0.5−−→PQ+10−−→AB−2−−→AC=→0.
b) Tính độ dài các vecto →u=→AB−25→AC→u=−−→AB−25−−→AC và →v=→AB+2→AC−→BC.→v=−−→AB+2−−→AC−−−→BC.
c) Chứng minh rằng đường thẳng PQPQ đi qua trọng tâm GG của tam giác ABC.ABC.
Quảng cáo
a) Từ giả thiết, sửu dụng quy tắc chèn điểm để biến đổi các vetco để chứng minh đẳng thức.
b) Biến đổi các vecto →u,→v→u,→v rồi tính độ dài các vetco.
c) Biểu diễn →GP,→QP−−→GP,−−→QP theo →AB,→AC.−−→AB,−−→AC.
Ba điểm G,P,QG,P,Q thẳng hàng ⇔→GP=k→QP(k≠0).⇔−−→GP=k−−→QP(k≠0).
a) Chứng minh rằng 5→PQ+10→AB−2→AC=→0.5−−→PQ+10−−→AB−2−−→AC=→0.
Ta có:
5→PQ+10→AB−2→AC=5→PA+5→AQ+10→AB−2→AC=5→AQ−5→AP+10→AB−2→AC=5.25→AC−5.2→AB+10→AB−2→AC=→0(dpcm).
b) Tính độ dài các vecto →u=→AB−25→AC và →v=→AB+2→AC−→BC.
Ta có: →u=→AB−25→AC=→AB−→AQ=→QB
⇒|→u|=|→QB|=QB
Áp dụng định lý Pitago cho ΔABQ vuông tại A ta có:
BQ2=AB2+AQ2=AB2+(25AC)2=22+(25.5)2=8⇒BQ=√8=2√2⇒|→u|=2√2.
→v=→AB+2→AC−→BC=→AB+2→AC−(→BA+→AC)=→AB+2→AC+→AB−→AC=2→AB+→AC=→AP+→AC=→AM⇒|→v|=AM.
Với M là điểm thỏa mãn APMC là hình chữ nhật.
⇒AM=PC (tính chất hình chữ nhật).
Áp dụng định lý Pitago cho ΔAPC vuông tại A ta có:
AP2=AP2+AC2=(2AB)2+AC2=(2.2)2+52=41⇒AP=√41⇒|→v|=√41.
c) Chứng minh rằng đường thẳng PQ đi qua trọng tâm G của tam giác ABC.
Vì G là trọng tâm ΔABC nên ta có: →GA+→GB+→GC=→0
→GA+→GA+→AB+→GA+→AC=→0⇔3→GA+→AB+→AC=→0⇔→GA=−13(→AB+→AC)
⇒→GP=→GA+→AP=−13(→AB+→AC)+2→AB=53→AB−13→AC.
→QP=→QA+→AP=−25→AC+2→AB
⇒→GP=56→QP
⇒G,P,Q thẳng hàng hay PQ đi qua trọng tâm G của ΔABC. (đpcm)
Tham Gia Group Dành Cho 2K9 Chia Sẻ, Trao Đổi Tài Liệu Miễn Phí
>> Học trực tuyến Lớp 10 cùng thầy cô giáo giỏi tại Tuyensinh247.com, Cam kết giúp học sinh học tốt, bứt phá điểm 9,10 chỉ sau 3 tháng, hoàn trả học phí nếu học không hiệu quả.
 |
 |
 |
 |
 |
 |
 |
 |
Hỗ trợ - Hướng dẫn
-
024.7300.7989
-
1800.6947
(Thời gian hỗ trợ từ 7h đến 22h)
Email: lienhe@tuyensinh247.com
