Tìm tham số $m$ để phương trình \(\sqrt {21 + 4x - {x^2}} - \dfrac{3}{4}x + 3 = m\left( {\sqrt {x + 3}

Câu hỏi số 720271:

Vận dụng cao

Tìm tham số $m$ để phương trình $\sqrt {21 + 4x - {x^2}} - \dfrac{3}{4}x + 3 = m\left( {\sqrt {x + 3} + 2\sqrt {7 - x} } \right)\,\,\,\left( * \right)$ có nghiệm thực.

A. 0

B. 1

C. 3

Đáp án đúng là: C

Quảng cáo

Xem lời giải

Câu hỏi:720271

Giải chi tiết

Điều kiện $- 3 \le x \le 7$ .

$\left( * \right) \Leftrightarrow 4\sqrt {21 + 4x - {x^2}} - 3x + 12 = 4m\left( {\sqrt {x + 3} + 2\sqrt {7 - x} } \right)\,\,\,\,\left( 1 \right)$

Đặt $t = \sqrt {x + 3} + 2\sqrt {7 - x} ,$ suy ra: ${t^2} - 19 = 4\sqrt {21 + 4x - {x^2}} - 3x + 12$

Ta có $t' = \dfrac{1}{{2\sqrt {x + 3} }} - \dfrac{1}{{\sqrt {7 - x} }}.$ Cho $t' = 0 \Leftrightarrow 2\sqrt {x + 3} = \sqrt {7 - x} \Leftrightarrow x = - 1$

Bảng biến thiên:

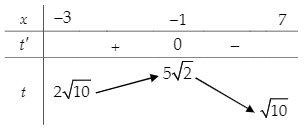
Dựa vào bảng biến thiên $\Rightarrow$ tập giá trị của $t$ là $t \in \left[ {\sqrt {10} ;5\sqrt 2 } \right]$

Khi đó $\left( 1 \right) \Leftrightarrow {t^2} - 19 = 4mt \Leftrightarrow 4m = \dfrac{{{t^2} - 19}}{t},\,\,\forall t \in \left[ {\sqrt {10} ;5\sqrt 2 } \right]$

Xét hàm số $f\left( t \right) = \dfrac{{{t^2} - 19}}{t}$ trên đoạn $\left[ {\sqrt {10} ;5\sqrt 2 } \right]$ có: $f'\left( t \right) = \dfrac{{{t^2} + 19}}{{{t^2}}} > 0,\,\,\forall t \in \left[ {\sqrt {10} ;5\sqrt 2 } \right]$

Suy ra hàm số $f\left( t \right)$ đồng biến trên đoạn $\left[ {\sqrt {10} ;5\sqrt 2 } \right]$

Do đó $\mathop {\min }\limits_{\left[ {\sqrt {10} ;5\sqrt 2 } \right]} f\left( t \right) = f\left( {\sqrt {10} } \right) = - \dfrac{{9\sqrt {10} }}{{10}}$ và $\mathop {\max }\limits_{\left[ {\sqrt {10} ;5\sqrt 2 } \right]} f\left( t \right) = f\left( {5\sqrt 2 } \right) = \dfrac{{31\sqrt 2 }}{{10}}$

Để $\left( * \right)$ có nghiệm $\Leftrightarrow \mathop {\min }\limits_{\left[ {\sqrt {10} ;5\sqrt 2 } \right]} f\left( t \right) \le 4m \le \mathop {\max }\limits_{\left[ {\sqrt {10} ;5\sqrt 2 } \right]} f\left( t \right) \Leftrightarrow - \dfrac{{9\sqrt {10} }}{{40}} \le m \le \dfrac{{31\sqrt 2 }}{{40}}$

Kết luận: Vậy $m \in \left[ { - \dfrac{{9\sqrt {10} }}{{40}};\dfrac{{31\sqrt 2 }}{{40}}} \right]$ thỏa mãn yêu cầu bài toán.

Vậy có 2 giá trị nguyên của $m \in \left\{ {0,1} \right\}$ thỏa mãn.

Group 2K8 ôn Thi ĐGNL & ĐGTD Miễn Phí

Câu hỏi trước Câu tiếp theo

>> Lộ Trình Sun 2025 - 3IN1 - 1 lộ trình ôn 3 kì thi (Luyện thi TN THPT & ĐGNL; ĐGTD - Click xem ngay) tại Tuyensinh247.com. Đầy đủ theo 3 đầu sách, Thầy Cô giáo giỏi, 3 bước chi tiết: Nền tảng lớp 12; Luyện thi chuyên sâu; Luyện đề đủ dạng đáp ứng mọi kì thi.